In previous studies, you may have developed formulas for finding the surface area of rectangular prisms, triangular prisms, cylinders, and pyramids. You may also have developed formulas for finding the volume of prisms, pyramids, cylinders, cones, and spheres.

In this lesson, you will focus on reading and interpreting problems involving surface area and volume. You will have to decide if the problem requires you to determine the surface area or the volume. You may also have to convert units within or between measurement systems in order to solve the problem.

Before you begin, recall the definitions of surface area and volume.

Surface area is a measure that tells you the number of square units it will take to completely cover the surfaces of a 3-dimensional figure. Surface area is an exterior attribute of a 3-dimensional figure; that is, it describes the size of the surfaces (outside) of the 3-dimensional figure. There are also several different types of surface area.

• Lateral surface area is the number of square units it will take to cover only the lateral surfaces of the 3-dimensional figure.
• Total surface area is the number of square units it will take to cover all of the surfaces of the 3-dimensional figure.

You may also be asked to determine the combined areas of only a few surfaces.

Volume is a measure that tells you the number of cubic units that will fit completely inside the 3-dimensional figure. Rectangular prisms can be used to show volume nicely, since they can be literally constructed out of smaller cubes.

When examining a problem, one of the first things that you need to determine is whether the problem is asking you to calculate the surface area or the volume of a 3-dimensional figure. Use the interactive below to practice sorting problems into surface area or volume problems.

Sometimes, when you are solving problems involving surface area or volume, you need to convert measurement units. It is very important to pay attention to the units of measurement that are being used in the problem and the units of measurement that are desired for the answer.

For each of the following unit conversions, drag the conversion factor from the box into the appropriate place in the equation. Next, perform the unit conversion in your notes. Check your answers for the unit conversions below the interactive.

1. A county highway crew stores sand in a cone-shaped silo for use during winter ice and snow storms. If the height of the cone is 15 feet and the diameter of the cone is 20 feet, how much sand can be stored in the silo?

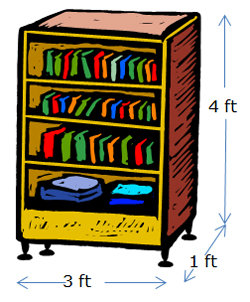2. Nedra has a bookcase that is shaped like a rectangular prism and will paint the left side, right side, and top red in order to match the colors in her bedroom. If the bookcase is 3 feet wide, 4 feet tall, and 1 foot deep, how much of the bookcase will be painted?

3. A wastebasket is in the shape of a cylinder. If the diameter of the wastebasket is 12 inches and the height is 12 inches, what is the maximum amount, in cubic feet, of waste that the wastebasket will hold?

4. Rafael has a soccer ball that is 9 inches in diameter. He wants to inflate the ball completely. How much air will the ball contain?

5. The Great Pyramid at Khufu, Egypt, is 146 meters tall and is a square pyramid with edge lengths along the base that are 230 meters in length. The slant height of each of the lateral faces is about 220 meters. If the lateral faces were originally covered with plaster, what is the amount of plaster that would be necessary for the Great Pyramid?

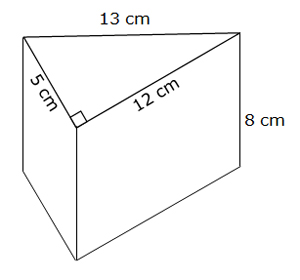6. A wedge of cheese is in the shape of a triangular prism. The base of the wedge is in the shape of a right triangle with a length of 5 centimeters and a width of 12 centimeters. The wedge itself is 8 centimeters tall. If the cheese is melted for a cheese sauce, how much molten cheese will there be in the bowl?