Learning Objectives
Learning Objectives
By the end of this section, you will be able to do the following:
- Distinguish between static friction and kinetic friction
- Solve problems involving inclined planes
| kinetic friction | static friction |
Static Friction and Kinetic Friction
Static Friction and Kinetic Friction
Recall from the previous chapter that friction is a force that opposes motion, and is around us all the time. Friction allows us to move, which you have discovered if you have ever tried to walk on ice.
There are different types of friction—kinetic and static. Kinetic friction acts on an object in motion, while static friction acts on an object or system at rest. The maximum static friction is usually greater than the kinetic friction between the objects.
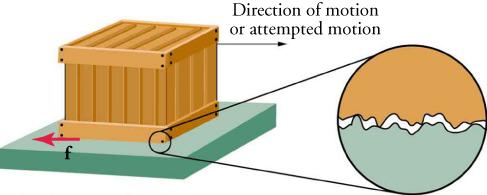
Imagine, for example, trying to slide a heavy crate across a concrete floor. You may push harder and harder on the crate and not move it at all. This means that the static friction responds to what you do—it increases to be equal to and in the opposite direction of your push. But if you finally push hard enough, the crate seems to slip suddenly and starts to move. Once in motion, it is easier to keep it in motion than it was to get it started because the kinetic friction force is less than the static friction force. If you were to add mass to the crate, (for example, by placing a box on top of it) you would need to push even harder to get it started and also to keep it moving. If, on the other hand, you oiled the concrete you would find it easier to get the crate started and keep it going.
Figure 5.35 shows how friction occurs at the interface between two objects. Magnifying these surfaces shows that they are rough on the microscopic level. So when you push to get an object moving (in this case, a crate), you must raise the object until it can skip along with just the tips of the surface hitting, break off the points, or do both. The harder the surfaces are pushed together (such as if another box is placed on the crate), the more force is needed to move them.
The magnitude of the frictional force has two forms: one for static friction, the other for kinetic friction. When there is no motion between the objects, the magnitude of static friction fs is
whereis the coefficient of static friction and N is the magnitude of the normal force. Recall that the normal force opposes the force of gravity and acts perpendicular to the surface in this example, but not always.
Since the symbolmeans less than or equal to, this equation says that static friction can have a maximum value ofThat is,
Static friction is a responsive force that increases to be equal and opposite to whatever force is exerted, up to its maximum limit. Once the applied force exceeds fs(max), the object will move. Once an object is moving, the magnitude of kinetic friction fk is given by
where is the coefficient of kinetic friction.
Friction varies from surface to surface because different substances are rougher than others. Table 5.2 compares values of static and kinetic friction for different surfaces. The coefficient of the friction depends on the two surfaces that are in contact.
| System | Static Friction | Kinetic Friction |
| Rubber on dry concrete | 1.0 | 0.7 |
| Rubber on wet concrete | 0.7 | 0.5 |
| Wood on wood | 0.5 | 0.3 |
| Waxed wood on wet snow | 0.14 | 0.1 |
| Metal on wood | 0.5 | 0.3 |
| Steel on steel (dry) | 0.6 | 0.3 |
| Steel on steel (oiled) | 0.05 | 0.03 |
| Teflon on steel | 0.04 | 0.04 |
| Bone lubricated by synovial fluid | 0.016 | 0.015 |
| Shoes on wood | 0.9 | 0.7 |
| Shoes on ice | 0.1 | 0.05 |
| Ice on ice | 0.1 | 0.03 |
| Steel on ice | 0.4 | 0.02 |
Since the direction of friction is always opposite to the direction of motion, friction runs parallel to the surface between objects and perpendicular to the normal force. For example, if the crate you try to push (with a force parallel to the floor) has a mass of 100 kg, then the normal force would be equal to its weight
perpendicular to the floor. If the coefficient of static friction is 0.45, you would have to exert a force parallel to the floor greater than
to move the crate. Once there is motion, friction is less and the coefficient of kinetic friction might be 0.30, so that a force of only 290 N
would keep it moving at a constant speed. If the floor were lubricated, both coefficients would be much smaller than they would be without lubrication. The coefficient of friction is unitless and is a number usually between 0 and 1.0.
Working with Inclined Planes
Working with Inclined Planes
We discussed previously that when an object rests on a horizontal surface, there is a normal force supporting it equal in magnitude to its weight. Up until now, we dealt only with normal force in one dimension, with gravity and normal force acting perpendicular to the surface in opposing directions (gravity downward, and normal force upward). Now that you have the skills to work with forces in two dimensions, we can explore what happens to weight and the normal force on a tilted surface such as an inclined plane. For inclined plane problems, it is easier breaking down the forces into their components if we rotate the coordinate system, as illustrated in Figure 5.36. The first step when setting up the problem is to break down the force of weight into components.
When an object rests on an incline that makes an anglewith the horizontal, the force of gravity acting on the object is divided into two components: A force acting perpendicular to the plane,, and a force acting parallel to the plane,. The perpendicular force of weight,, is typically equal in magnitude and opposite in direction to the normal force,The force acting parallel to the plane,, causes the object to accelerate down the incline. The force of friction,, opposes the motion of the object, so it acts upward along the plane.
It is important to be careful when resolving the weight of the object into components. If the angle of the incline is at an angleto the horizontal, then the magnitudes of the weight components are
Instead of memorizing these equations, it is helpful to be able to determine them from reason. To do this, draw the right triangle formed by the three weight vectors. Notice that the angle of the incline is the same as the angle formed betweenand. Knowing this property, you can use trigonometry to determine the magnitude of the weight components
Watch Physics
Inclined Plane Force Components
This video shows how the weight of an object on an inclined plane is broken down into components perpendicular and parallel to the surface of the plane. It explains the geometry for finding the angle in more detail.
This video shows how the weight of an object on an inclined plane is broken down into components perpendicular and parallel to the surface of the plane. It explains the geometry for finding the angle in more detail.
When the surface is flat, you could say that one of the components of the gravitational force is zero; Which one? As the angle of the incline gets larger, what happens to the magnitudes of the perpendicular and parallel components of gravitational force?
- When the angle is zero, the parallel component is zero and the perpendicular component is at a maximum. As the angle increases, the parallel component decreases and the perpendicular component increases. This is because the cosine of the angle shrinks while the sine of the angle increases.
- When the angle is zero, the parallel component is zero and the perpendicular component is at a maximum. As the angle increases, the parallel component decreases and the perpendicular component increases. This is because the cosine of the angle increases while the sine of the angle shrinks.
- When the angle is zero, the parallel component is zero and the perpendicular component is at a maximum. As the angle increases, the parallel component increases and the perpendicular component decreases. This is because the cosine of the angle shrinks while the sine of the angle increases.
- When the angle is zero, the parallel component is zero and the perpendicular component is at a maximum. As the angle increases, the parallel component increases and the perpendicular component decreases. This is because the cosine of the angle increases while the sine of the angle shrinks.
Tips For Success
Normal force is represented by the variableThis should not be confused with the symbol for the newton, which is also represented by the letter N. It is important to tell apart these symbols, especially since the units for normal force () happen to be newtons (N). For example, the normal force,, that the floor exerts on a chair might beOne important difference is that normal force is a vector, while the newton is simply a unit. Be careful not to confuse these letters in your calculations!
To review, the process for solving inclined plane problems is as follows:
- Draw a sketch of the problem.
- Identify known and unknown quantities, and identify the system of interest.
- Draw a free-body diagram (which is a sketch showing all of the forces acting on an object) with the coordinate system rotated at the same angle as the inclined plane. Resolve the vectors into horizontal and vertical components and draw them on the free-body diagram.
- Write Newton’s second law in the horizontal and vertical directions and add the forces acting on the object. If the object does not accelerate in a particular direction (for example, the x -direction) then Fnet x = 0. If the object does accelerate in that direction, Fnet x = ma.
- Check your answer. Is the answer reasonable? Are the units correct?
Worked Example
Finding the Coefficient of Kinetic Friction on an Inclined Plane
A skier, illustrated in Figure 5.37(a), with a mass of 62 kg is sliding down a snowy slope at an angle of 25 degrees. Find the coefficient of kinetic friction for the skier if friction is known to be 45.0 N.
Strategy
The magnitude of kinetic friction was given as 45.0 N. Kinetic friction is related to the normal force N as . Therefore, we can find the coefficient of kinetic friction by first finding the normal force of the skier on a slope. The normal force is always perpendicular to the surface, and since there is no motion perpendicular to the surface, the normal force should equal the component of the skier’s weight perpendicular to the slope.
That is,
Substituting this into our expression for kinetic friction, we get
which can now be solved for the coefficient of kinetic friction μk.
Solving forgives
Substituting known values on the right-hand side of the equation,
This result is a little smaller than the coefficient listed in Table 5.1 for waxed wood on snow, but it is still reasonable since values of the coefficients of friction can vary greatly. In situations like this, where an object of mass m slides down a slope that makes an angle θ with the horizontal, friction is given by
Worked Example
Weight on an Incline, a Two-Dimensional Problem
The skier’s mass, including equipment, is 60.0 kg. (See Figure 5.38(b).) (a) What is her acceleration if friction is negligible? (b) What is her acceleration if the frictional force is 45.0 N?
Strategy
The most convenient coordinate system for motion on an incline is one that has one coordinate parallel to the slope and one perpendicular to the slope. Remember that motions along perpendicular axes are independent. We use the symbolto mean perpendicular, andto mean parallel.
The only external forces acting on the system are the skier’s weight, friction, and the normal force exerted by the ski slope, labeled,, andin the free-body diagram.is always perpendicular to the slope andis parallel to it. Butis not in the direction of either axis, so we must break it down into components along the chosen axes. We defineto be the component of weight parallel to the slope andthe component of weight perpendicular to the slope. Once this is done, we can consider the two separate problems of forces parallel to the slope and forces perpendicular to the slope.
The magnitude of the component of the weight parallel to the slope is, and the magnitude of the component of the weight perpendicular to the slope is
(a) Neglecting friction: Since the acceleration is parallel to the slope, we only need to consider forces parallel to the slope. Forces perpendicular to the slope add to zero, since there is no acceleration in that direction. The forces parallel to the slope are the amount of the skier’s weight parallel to the slopeand friction. Assuming no friction, by Newton’s second law the acceleration parallel to the slope is
Where the net force parallel to the slope, so that
is the acceleration.
(b) Including friction: Here we now have a given value for friction, and we know its direction is parallel to the slope and it opposes motion between surfaces in contact. So the net external force is now
and substituting this into Newton’s second law,gives
We substitute known values to get
or
which is the acceleration parallel to the incline when there is 45 N opposing friction.
Since friction always opposes motion between surfaces, the acceleration is smaller when there is friction than when there is not.
Practice Problems
When an object sits on an inclined plane that makes an angle θ with the horizontal, what is the expression for the component of the objects weight force that is parallel to the incline?
Snap Lab
Friction at an Angle: Sliding a Coin
An object will slide down an inclined plane at a constant velocity if the net force on the object is zero. We can use this fact to measure the coefficient of kinetic friction between two objects. As shown in the first Worked Example, the kinetic friction on a slope, and the component of the weight down the slope is equal to. These forces act in opposite directions, so when they have equal magnitude, the acceleration is zero. Writing these out
Solving for, sincewe find that
- 1 coin
- 1 book
- 1 protractor
- Put a coin flat on a book and tilt it until the coin slides at a constant velocity down the book. You might need to tap the book lightly to get the coin to move.
- Measure the angle of tilt relative to the horizontal and find.
True or False—If only the angles of two vectors are known, we can find the angle of their resultant addition vector.
- True
- False
Check Your Understanding
Check Your Understanding
- Friction is an internal force that opposes the relative motion of an object.
- Friction is an internal force that accelerates an object’s relative motion.
- Friction is an external force that opposes the relative motion of an object.
- Friction is an external force that increases the velocity of the relative motion of an object.
- Kinetic and static friction both act on an object in motion.
- Kinetic friction acts on an object in motion, while static friction acts on an object at rest.
- Kinetic friction acts on an object at rest, while static friction acts on an object in motion.
- Kinetic and static friction both act on an object at rest.
- The kinetic friction has a greater value because the friction between the two surfaces is more when the two surfaces are in relative motion.
- The static friction has a greater value because the friction between the two surfaces is more when the two surfaces are in relative motion.
- The kinetic friction has a greater value because the friction between the two surfaces is less when the two surfaces are in relative motion.
- The static friction has a greater value because the friction between the two surfaces is less when the two surfaces are in relative motion.